The eye can function over a large range of luminance levels; it must also be able to handle the different rates of change in luminance. Our eyes are constantly sampling information of images projected onto the retina in a periodic manner. Information is then integrated so objects around us appear to be stable or move smoothly. Since there is a finite amount of time required to collect and process information, there are limitations to the responsiveness of our visual system to rates of change. When intermittent stimuli are presented to the eye they are perceived as separate if the rate at which they are presented is below a certain value. If the rate of presentation of the intermittent stimuli is slow, it appears to stay on but with changes in intensity, producing the sensation called flicker. Above a certain critical rate, the flicker ceases. This point is called the critical flicker frequency and is influenced by a number of factors. The first factor to be considered is temporal summation properties of the visual system.
1. Temporal Summation.
Temporal resolution and temporal summation.
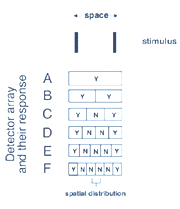
In the spatial domain, detection of two lights in space requires the appropriate detector array (figure 1). In order for us to discriminate the two lines, a response given by detector array C to F is required. All these detector arrays provide a Yes-No-Yes response and thus allow the discrimination of the two lines.
Fig. 1. Detector array C and beyond allows the detection of the two lines
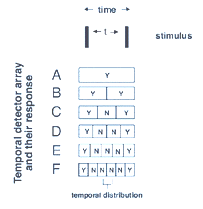
In the temporal domain, the same principle applies, except now, the stimulus is separated in time (figure 2). The separation between the two lines is in the temporal domain (two flashes are delivered) after a time interval t. The detector array now has different temporal integration times. For example, detector A integrates over time = t, whereas detector array B has an integration time of time = 0.5t, array C, time = 0.33t and so on. Because of the shorter integration time for detector array C and beyond, such an array will be able to discriminate the two flashes that are separated by an interval of t.
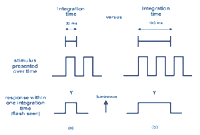
To detect a flash of light one after the other, an appropriate integration time is required (figure 3). The period of integration is up to 0.1 seconds or 100 ms (for rods) and 10 to 15 ms for cones. The advantage of long integration time is that under limited light level conditions, threshold will be reached, whereas when light levels are not limiting (cone or photopic vision), a short integration time is preferable to improve temporal resolution.
Temporal integration time is related to temporal summation. Temporal summation refers to the eye’s ability to sum the effects of individual quanta of light over time. However, temporal summation only occurs within a certain period of time, called the critical duration or critical period. According to Bloch’s law of vision, within this critical duration, threshold is reached when the total luminous energy is reached. Bloch’s Law of temporal summation is analogous to Ricco’s Law of spatial summation. Bloch’s law states that total luminous energy is a constant value (k), thus threshold is reached when the product of luminance (L) and stimulus duration (t) equals this constant. In other words, when luminance is halved, a doubling in stimulus duration is required to reach threshold. When luminance is doubled, threshold can be reached in half the duration. Bloch’s Law is expressed as:
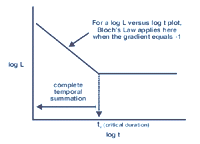
L. tn = k where L is the luminance of the stimulus, t is the duration of the stimulus, k is a constant value and n describes whether temporal summation is complete (n=1) or partial (0<n<1). No temporal summation occurs when n = 0 (figure 4).
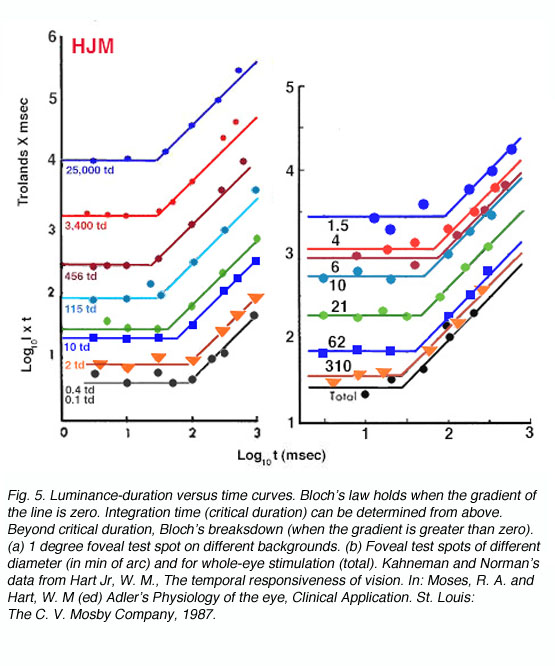
Fig. 4. Temporal summation data plotted as log L vs log t, showing where Bloch’s Law applies
Critical duration is shorter for stimulus of high luminance as threshold is reached faster and slower for stimulus of low luminance as a longer period of time is required to sum the quanta to reach threshold. Temporal summation ceases beyond the temporal integration time. Above this value, threshold is dependent only on luminance rather than the product of luminance and duration.
Temporal summation is also affected by other test variables such as background luminance and the size of the stimulus size. Critical duration is longer for brighter background and smaller test stimuli. When temporal summation data is plotted as log L.t versus log t rather than log L versus log t (as in figure 4), the slope of zero identifies Bloch’s Law (figure 5).
The above plots show that temporal summation is longer for low light levels indicating the larger temporal summation characteristics for scotopic vision. As light intensity is increased, eg, 25,000 trolands, the critical duration is of the order of 20-30msec. The interrelationship between temporal integration and spatial summation is shown on the right panel where Bloch’s law is measured for different size test stimuli. Decreasing test size results in increased temporal summation, and hence poorer temporal discrimination. We will investigate this phenomenon further when we review flicker discrimination for different sized stimuli in the next section.
Broca-Sulzer effect.
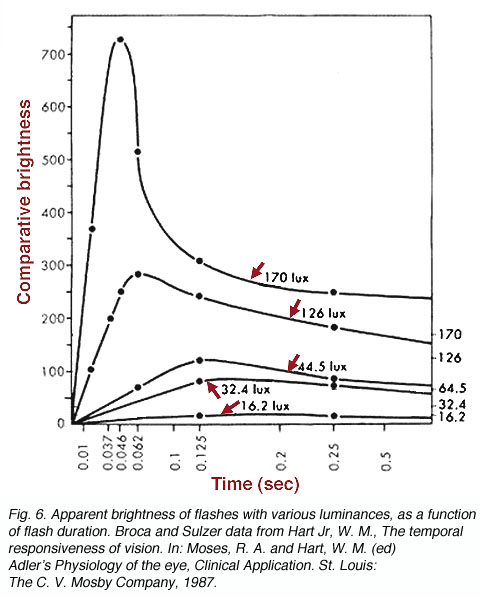
In addition to basic discrimination characteristics of temporal resolution, there are several interesting perceptual phenomena. One of these phenomena is the Broca-Sulzer effect that describes the apparent transient increase in brightness of a flash of short duration. Subjective flash brightness occur with flash duration of 50 to 100 milliseconds. This phenomenon is associated with temporal summation and explains the levelling off of brightness to a plateau. When the light is turned on, time is required for temporal summation to reach threshold for light of low luminance. Light of high luminance reach this threshold very quickly. As flash duration increases, brightness levels off to a plateau as temporal summation begins to breakdown according to Bloch’s law after the critical duration. The apparent transient peak in brightness is probably due to an underlying neural mechanism (figure 6).
2. Critical flicker frequency (CFF).
Critical flicker frequency (CFF) is the transition point of an intermittent light source where the flickering light ceases and appears as a continuous light. There are a multitude of factors that determine our perception of flicker that includes the intensity and size of the test stimulus.
Factors affecting CFF.
The Ferry-Porter Law states that CFF is proportional to the logarithm of the luminance of flickering stimulus (L). It can be expressed as
CFF = a logL + b
Where a and b are constants. With foveal observation, this relationship holds over a wide range (0.5 to 10,000 trolands; figure 7). The above equation implies that when CFF is plotted as a function of log L, a straight line will identify the region where the Ferry-Porter Law holds. As the intensity of the test stimulus is increased, our perception of flicker also increases. From a practical point of view, if a stimulus is flickering, such as computer monitor, decreasing the intensity level will eliminate the flicker.
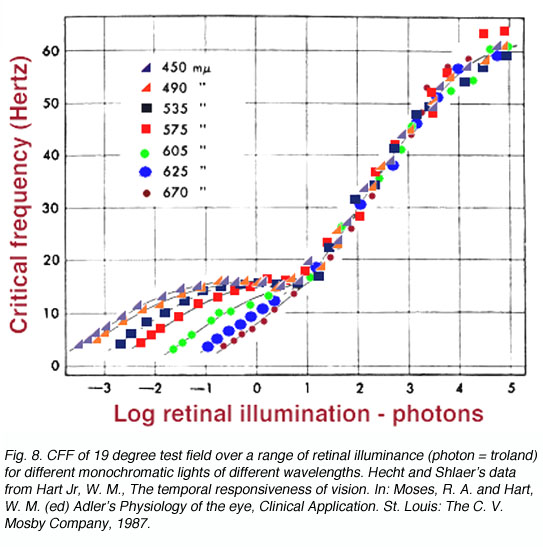
Spectral Composition: Under photopic levels, lights of different wavelengths, when adjusted to match them for brightness, conforms to the Ferry-Porter Law, and follows the logarithmic function as brightness increases. However, under scotopic levels (rods functioning), the wavelengths fan out (figure 8). This is due to the different spectral sensitivity of the scotopic system to the photopic system. If plotted as scotopic photons, the bottom part of the curve would collapse into one and appear like the 19o curve in figure 10. Note that the temporal resolution to short wavelength test stimuli is different. However, in general terms, the plot below is correct although the units chosen for the scotopic component (lower branches) give the misconception that temporal resolution is different for the rod system.An important aspect of cone vision is that when the short-wavelength pathway is isolated (Kelly, 1974), the temporal resolution is lower, close to 10-15 Hz, rather than the closer to 60 Hz for the longer wavelength pathways. This general phenomenon, is characteristic of the short-wavelength pathway that is known to have larger spatial summation approximately 15’ at about one degree eccentricity (the location of high S-cone density), whereas the longer wavelength pathways have a spatial summation of 4’ (Brindley, 1954). In the temporal domain, at high light levels, the S-cone pathways has a temporal summation time of approximately 100ms, whereas the longer-wavelength cone pathway has a temporal integration of approximately 50msec (Krauskopf and Mollon, 1971).
Retinal Position: Since the CFF is different for rod and cones, the CFF for the test field will depend on the proportion of rods and cones being stimulated. Because the proportion of rod and cones change with eccentricity, a foveal test stimulus will follow the Ferry-Porter Law and show no kink (one branch only) in the curve since only cones are present at the fovea. An extrafoveal test stimulus will show a kink (two branches) in the CFF function since the rods determine the CFF at low retinal illuminances and the cones determining CFF at higher retinal illuminance. Note that the Ferry-Porter Law applies over a decreasing range as the eccentricity increases and that temporal resolution is poorer for eccentric locations (figure 9).
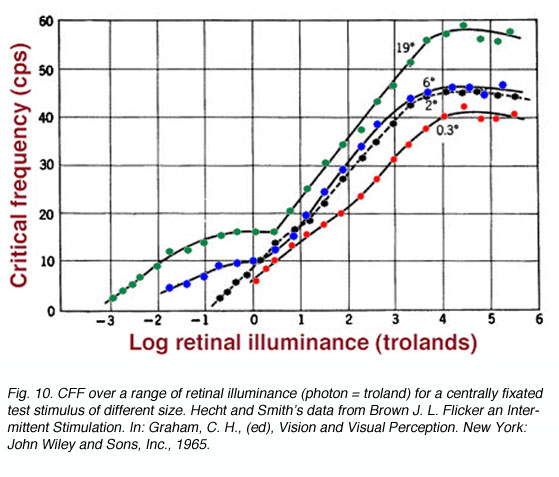
The Ferry-Porter law has been further examined for a single cone type, using conditions that eliminate detection of the flickering stimulus by rods. Under these conditions, the law has been found to hold despite changes in stimulus size or modulation amplitude (Tyler and Hamer, 1990). However, the slope of the Ferry-Porter law changes with eccentricity, becoming steeper with eccentrically presented targets. This latter finding is consistent with previous work suggesting that there is an increase in the speed of photopic retinal responses in the periphery, once stimuli have been appropriately scaled (Tyler, 1985). This increase in speed has been hypothesized to relate to the change in cone photoreceptor outer-segment length that occurs in the periphery (Tyler, 1985). Such scaling has not been performed to the classic data sets presented here. Size of Test Field: Due to the different population of rods and cones in the retina and different spatial summation properties, CFF will be dependent on the area of the retina being stimulated. Instead of varying retinal eccentricity as above, the size of the centrally fixated test field is varied (figure 10). As the test field increases, two branches begin to appear. The lower branch representing rod function. The maximum CFF, and hence maximum temporal resolution is achieved by large test targets that have the shortest integration time noted earlier.
The Talbot-Plateau Law describes the brightness of an intermittent light source which has a frequency above the CFF. This law states that above CFF, subjectively fused intermittent light and objectively steady light (of equal colour and brightness) will have exactly the same luminance. In another words, brightness sensation from the intermittent light source is the same as if the light perceived during the various periods of stimulation had been uniformly distributed over the whole time. The Talbot-Plateau Law applies only above the CFF.
The Brücke-Bartley (brightness enhancement) effect is a phenomenon related to the Broca-Sulzer effect. When the frequency is gradually lowered below the CFF, the effective brightness of the test field begins to rise. Not only does the brightness reach a value equal to that of the uninterrupted light, but even transcends it, reaching a maximum when the flash rate is about 8 to 10 Hz.
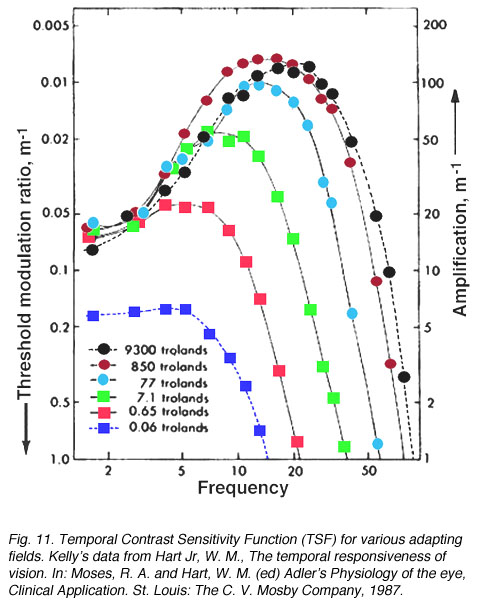
3. Temporal Contrast Sensitivity.
In the spatial domain, spatial vision can be characterised by the contrast sensitivity function (CSF). To thoroughly investigate the visual system to flicker, a Temporal Contrast Sensitivity Function (TSF) or a de Lange Function can be plotted (De Lange, 1958). A TSF is a plot of how flicker varies with contrast and vice versa. Above the curve represents no flicker while flicker can be detected below the curve (figure 11). The eye appears to be most sensitive to a frequency of 15 to 20 Hz at high luminances (photopic vision). At photopic light levels, less than 1% contrast is required to detect the stimulus and the high temporal frequency cut off is close to 60 Hz. At low light levels the maximum contrast is about 20% and the high temporal frequency cut off is approximately 15 Hz. To detect flicker of high frequencies, maximum contrast is required. Temporal resolution is not as efficient at low luminances (scotopic vision).
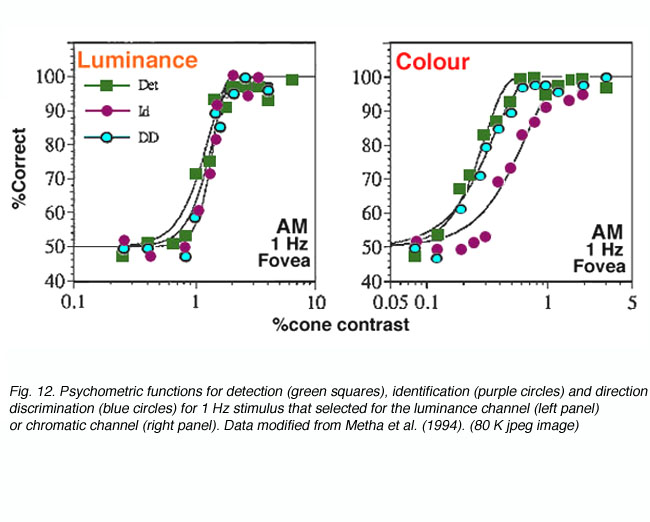
Different sensitivity profiles exist for the different components of the visual system (opponent versus non-opponent), to discriminate motion. For the luminance system, detection, identification and direction discrimination provide equivalent thresholds. However, at all eccentricities tested, the chromatic system required approximately 0.3 log units higher contrast levels to signal direction of motion (figure 12: Metha et al., 1994). Overall, these results imply that both luminance (non-opponent) and chromatic (opponent) visual channels are involved in motion discrimination.
References.
Brown JL. Flicker: an intermittent dtimulation. In: Graham CH, editor. Vision and visual perception. New York: John Wiley and Sons, Inc., 1965.
Brindley GS. The summation areas of human colour-receptive mechanisms at increment threshold. J Physiol. 1954;124:400–408. [PubMed]
De Lange DZN H. Research into the dynamic nature of the human fovea–cortex systems with intermittent and modulated light. II. Phase shift in brightness and delay in color perception. J Opt Soc Am. 1958;48:784–789. [PubMed]
Hart WM Jr. The temporal responsiveness of vision. In: Moses RA, Hart WM, editors. Adler’s physiology of the eye. Clinical application. St. Louis (MO): The C. V. Mosby Company; 1987.
Kelley DH. Spatio-temporal frequency characteristics of color-vision mechanisms. J Opt Soc Am. 1974;64:983–990. [PubMed]
Krauskopf J, Mollon JD. The independence of the temporal integration properties of individual chromatic mechanism in the human eye. J Physiol. 1971;219:611–623. [PubMed] [Free Full text in PMC]
Metha AB, Vingrys AJ, Badcock DR. Detection and discrimination of moving stimuli: the effects of color, luminance, and eccentricity. J Opt Soc Am A Opt Image Sci Vis. 1994;11:1697–1709. [PubMed]
Tyler CW. Analysis of visual modulation sensitivity. II. Peripheral retina and the role of photoreceptor dimensions. J Opt Soc Am A. 1985;2:393–398. [PubMed]
Tyler CW, Hamer RD. Analysis of visual modulation sensitivity. IV. Validity of the Ferry-Porter law. J Opt Soc Am A. 1990;7:743–759. [PubMed]
Last Update: June 5, 2007.
The author
Michael Kalloniatis was born in Athens Greece in 1958. He received his optometry degree and Master’s degree from the University of Melbourne. His PhD was awarded from the University of Houston, College of Optometry, for studies investigating colour vision processing in the monkey visual system. Post-doctoral training continued at the University of Texas in Houston with Dr Robert Marc. It was during this period that he developed a keen interest in retinal neurochemistry, but he also maintains an active research laboratory in visual psychophysics focussing on colour vision and visual adaptation. He was a faculty member of the Department of Optometry and Vision Sciences at the University of Melbourne until his recent move to New Zealand. Dr. Kalloniatis is now the Robert G. Leitl Professor of Optometry, Department of Optometry and Vision Science, University of Auckland. e-mail: m.kalloniatis@unsw.edu.au
Charles Luu was born in Can Tho, Vietnam in 1974. He was educated in Melbourne and received his optometry degree from the University of Melbourne in 1996 and proceeded to undertake a clinical residency within the Victorian College of Optometry. During this period, he completed post-graduate training and was awarded the post-graduate diploma in clinical optometry. His areas of expertise include low vision and contact lenses. During his tenure as a staff optometrist, he undertook teaching of optometry students as well as putting together the “Cyclopean Eye”, in collaboration with Dr Michael Kalloniatis. The Cyclopean Eye is a Web based interactive unit used in undergraduate teaching of vision science to optometry students. He is currently in private optometric practice as well as a visiting clinician within the Department of Optometry and Vision Science, University of Melbourne.